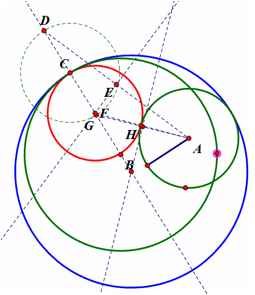
To start this exploration I began with two circles, one inside the other
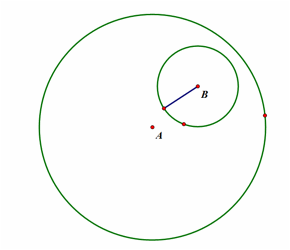
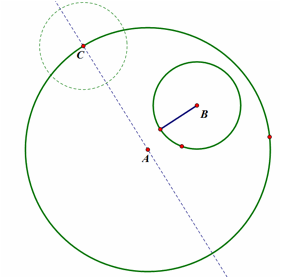
Then, I selected a point on Circle A and created a new circle C centered at the point with the same radius as Circle B;
I used the point where the new Circle C intersected with the Line A to create a line segment from D to B, and found the midpoint, E.
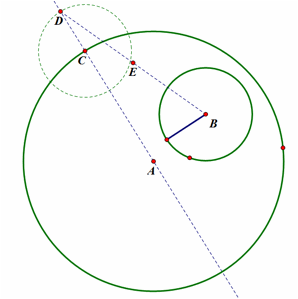
Line segment DB will be the base of the isosceles triangle whose perpendicular bisector will pass through the center of the tangent circle.
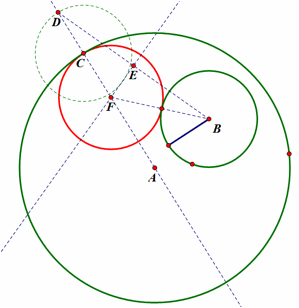
The tangent circle is in red and constructed with center F passing through the point C.
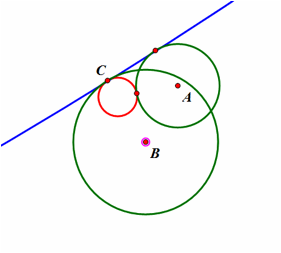
Here is what happens when the original circles overlap and when the circles are disjoint:
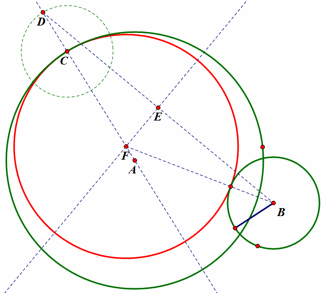
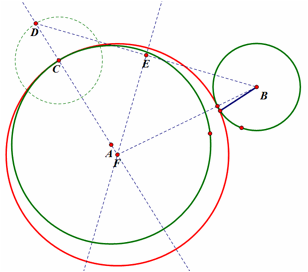
But there is another tangent circle to consider constructed below in blue using the interior intersection point of Circle C with Line B.
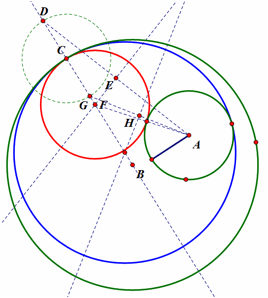
This is what happens when the two original circles (in green) overlap and when they are disjoint:
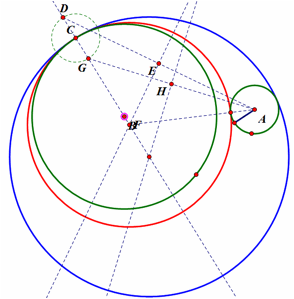
Here is a “cleaned up” version of the two tangent circles, in red and blue:
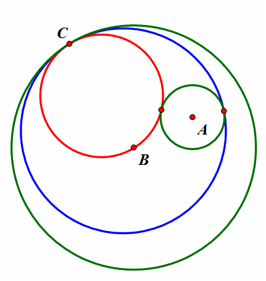
A couple interesting things happened while I was “dragging” the circles around…
Where did the blue tangent circle go?
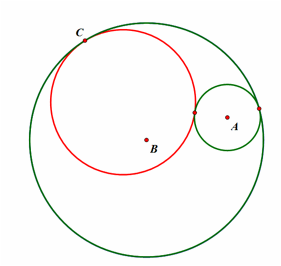
And the blue “circle” does not seem to be a circle anymore here…